| Скачать .docx |
Реферат: Решения к Сборнику заданий по высшей математике Кузнецова Л.А. - 2. Дифференцирование. Зад.15
Задача 15
. Найти производную ![]() .
.
15.1. 
x'= 6t*t3 -3t2 (3t2 +1) = -t2 -1
3t6 t4
y'= cos(t3 /3+t)(t2 +1)
y'x = cos(t3 /3+t)(t2 +1)t4 = -t4 cos(t3 /3+t)
-t2 -1
15.2. 
x'= -t _
√(1-t2)
y'= 1 _
2√(1+t)cos2 √(1+t)
y'x = -√(1-t2 ) = -√(1-t2 ) _
2t√(1+t)cos2 √(1+t) 2tcos2 √(1+t)
15.3. 
x'= 1-t _
√(2t-t2 )
y'= 2 _
3 3 √(1-t)5
y'x = 2√(2t-t2 ) = 2√(2t-t2 ) _
3 3 √(1-t)5 (1-t) 3 3 √(1-t)2 (1-t)2
15.4. 
x'= cost = 1
√(1-sin2 t)
y'= sint = 1
√(1-cos2 t)
y'x = 1
15.5. 
x'= 1+t/√(t2 +1) = 1 _
t+√(t2 +1) √(t2 +1)
y'= √(t2 +1)+ t2 = 2t2 +1_
√(t2 +1) √(t2 +1)
y'x = (2t2 +1)√(t2 +1) = 2t2 +1
√(t2 +1)
15.6. 
x'= 1-t _
√(2t-t2 )
y'= 1 = 1 _
√(1-(t-1)2 ) √(2t-t2 )
y'x = √(2t-t2 ) = 1_
√(t2-t2 )(1-t) 1-t
15.7. 
x'= -2et _ = -2et _
sin2 (2et ) 4sin2 et cos2 et
y'= et = et _
tget cos2 et sinet coset
y'x = 4et sin2 et cos2 et = -2sinet coset
-2et sinet coset
15.8. 
x'= -1 = -1 _
ctgt sin2 t sint cost
y'= 2sint
cos3 t
y'x = -cos3 t = -1/2*ctg2 t
2sin2 tcost
15.9. 
x'= et/2 _
2(1+et )
y'= et _
2√(1+et )
y'x = 2et (1+et ) = √(et +e2t )
2et/2 √(et +1)
15.10. 
x'= √(1+t) *√(1+t) *-1-t-1+t = -1_
√(1-t) 2√(1-t) (1+t)2 1-t2
y'= -t _
√(1-t2 )
y'x = t(1-t2 ) = t√(1-t2 )
√(1-t2 )
15.11. 
x'= 2t3_
1-t4
y'= (1+t2 )(-2t(1+t2 )-2t(1-t2 )) = -2
√(1+2t2 +t4 -1+2t2 -t4 )(1+t2 )
y'x = -2(1-t4 ) = t4 -1
2t3 t3
15.12. 
x'= -t _
√(1-t2 )
y'= √(1-t2 )+t2 /√(1-t2 ) = 1 _
1-t2 (1-t2 )3/2
y'x = -√(1-t2 )_ = 1_
t(1-t2 )3/2 t3 -t
15.13. 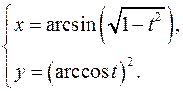
x'= -t = -1 _
√(1-1+t2 )√(1-t2 ) √(1-t2 )
y'= -2arccost
√(1-t2 )
y'x = 2arccost√(1-t2 ) = 2arccost
√(1-t2 )
15.14. 
x'= √(1-t2 )+t2 /√(1-t2 ) = 1 _
1-t2 (1-t2 )3/2
y'= t *-t2 /√(1-t2 )-1-√(1-t2 ) = -1
1+√(1-t2 ) t2 t
y'x = √(1-t2 )
t2
15.15. 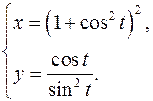
x'= -4(1+cos2 t)costsint
y'= -sin3 t-2cos2 tsint = -1-cos2 t
sin4 t sin3 t
y'x = 1+cos2 t = 1 _
4sin3 t(1+cos2 t)costsint 4sin4 tcost
15.16. 
x'= (1+t)(-1-t-1+t) = -2_
(1-t)(1+t)2 1-t2
y'= -t _
√(1-t2 )
y'x = -t (1-t2 )_ = t√(1-t2 )
-2√(1-t2 ) 2
15.17. 
x'= 1 = 1 _
t2 √(1-1/t2 ) t√(t2 -1)
y'= t + t = 2t _
√(t2 -1) √(t2 -1) √(t2 -1)
y'x = 2t2 √(t2 -1) = 2t2
√(t2 -1)
15.18. 
x'= 1_
tln2 t
y'= t *-t2 /√(1-t2 )-1-√(1-t2 ) = -1
1+√(1-t2 ) t2 t
y'x = -tln2 t = -ln2 t
t
15.19. 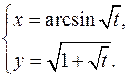
x'= 1 _
2√t√(1-t)
y'= 1 _
4√t√(1+√t)
y'x = 2√t√(1-t) = √(1-√t)
4√t√(1+√t) 2
15.20. 
x'= 2arcsint
√(1-t2 )
y'= √(1-t2 )+t2 /√(1-t2 ) = 1 _
1-t2 (1-t2 )3/2
y'x = √(1-t2 ) = 1 _
2(1-t2 )3/2 arcsint 2(1-t2 )arcsint
15.21. 
x'= √(t2 +1)+t2 /√(t2 +1) = 2t2 +1
√(t2 +1)
y'= t *-t2 /√(1-t2 )-1-√(1-t2 ) = -1
1+√(1-t2 ) t2 t
y'x = -√(t2 +1) = -1 _
(2t2 +1)√(t2 +1) 2t2 +1
15.22. 
x'= 1/(1+t2 )
y'= (t+1)(t(t+1)/√(t2 +1)-√(1+t2 )) = t-1 _
√(1+t2 )(1+t) √(t2 +1)(1+t)
y'x = (t-1)(1+t2 ) = (t-1)√(1+t2 )
√(1+t2 )(t+1) 1+t
15.23. 
x'= -2t/(1-t2 )
y'= -t = -1/√(1-t2 )
√(1-1+t2 )√(1-t2 )
y'x = 1-t2 = √(1-t2 )
2t√(1-t2 ) 2t
15.24. 
x'= (t-1)2 (t-1-t-1) = -1_
((t-1)2 +(t+1)2 )(t-1)2 t2 +1
y'= -t = -1/√(1-t2 )
√(1-1+t2 )√(1-t2 )
y'x = t2 +1_
√(1-t2 )
15.25. 
x'= √(1+sint)√(1+sint)(-cost(1+sint)-cost(1-sint)) = -1_
2√(1-sint)√(1-sint)(1+sint)2 cost
y'= tgt/cos2 t-tgt= tg3 t
y'x = -tg3 tcost
15.26. 
x'= 1-2t _ t√t(-t-1+t) = √(1-t)
2√(t-t2 ) 2(t+1-t)√(1-t)t2 2√t
y'= 1 + arcsin√t _ √(1-t) = arcsin√t
2√t 2√(1-t) 2√t√(1-t) 2√(1-t)
y'x = 2√t arcsin√t = √t arcsin√t
2(1-t) (1-t)
15.27. 
x'= 1 = 1 _
tgtcos2 t sintcost
y'= -2cost
sin3 t
y'x = -2cost = -2_
sin4 tcost sin4 t
15.28. 
x'= (2tlnt+t)(1-t2 )+2t3 lnt – 2t = 2tlnt
(1-t2 )2 2√(1-t2 ) (1-t2 )
y'= √(1-t2 )+t2 /√(1-t2 ) arcsint + t/(1-t2 ) – t/(1-t2 ) = arcsint
1-t2 (1-t2 )3/2
y'x = arcsint(1-t2 )2 = arcsint√(1-t2 )
2tlnt(1-t2 )3/2 2tlnt
15.29. 
x'= 2esec^2t sec2 t tgt= 2esec^2t sint
cos3 t
y'= lncost _ sint + 1/cos2 t-1= lncost-sintcost+sin2 t
cos2 t cost cos2 t
y'x = 1/2*e-sec^2t ctgt(lncost-sintcost+sin2 t)
15.30. 
x'= √(1-t2 )+t2 /√(1-t2 ) arcsint + t/(1-t2 ) – t/(1-t2 ) = arcsint
1-t2 (1-t2 )3/2
y'= √(1-t2 )+t2 /√(1-t2 ) = 1 _
1-t2 (1-t2 )3/2
y'x = (1-t2 )3/2 = 1 _
(1-t2 )3/2 arcsint arcsint
15.31. 
x'= 1+t/√(t2 +1) = 1 _
t+√(t2 +1) √(t2 +1)
y'= t _ t *-t2 /√(1-t2 )-1-√(1-t2 ) = t + 1 = t2 +2√(1-t2 )
2√(1+t2 ) 1+√(1-t2 ) t2 2√(1+t2 ) t 2t√(1-t2 )
y'x = (t2 +2√(1-t2 ))√(1+t2 )
2t√(1-t2 )